Friday, August 3, 2018

STRENGTH OF MATERIALS

An aluminum alloy [E = 8900 ksi] pipe with a cross-sectional area of A1 = 4.57 in.2 is connected at flange Bto a steel [E = 26600 ksi] pipe with a cross-sectional area of A2 = 3.08 in.2. The assembly is connected to rigid supports at Aand C. Assume L1=168 in., L2=228 in., and P=52 kips. For the loading shown, determine:(a) the normal stresses in aluminum pipe (1) and steel pipe (2) (,  use positive if tensile, negative if compressive).(b) the deflection uB (use positive if it moves to the right and negative if it moves to the left) of flange B.

? RA-2p

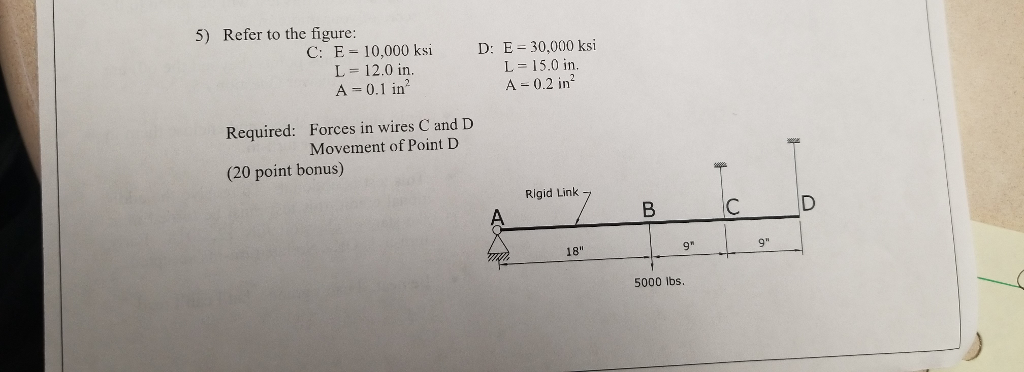
CALCULATE THE FORCE IN THE CABLE C AND D, AND EXTENSION IN CABLE D.

5) Refer to the figure: C: E 10,000 ksi D: E 30,000 ksi L 12.0 in A 0.1 in L = 15.0 in A 0.2 in Forces in wires C and D Movement of Point D Required: (20 point bonus) Rigid Link 18 5000 lbs.

Steps to solve:

  1. Draw FBD
  2. Taking moment about A
  3. Use triangle properties and find relation.
  4. Now find force in member C and D.
  5. finally calculate the movement of D using Axial deflection formula.




CALCULATE THE FORCE IN CABLE AT B AND DIAMETER OF CABLE

concept checkpoints Determine the internal axial force in segment (2) of the compound axial assembly. Give your answer in units of kN. 30 kN 75 kN 50 kN 30 kN enter

Steps to solve:

  1. Draw FBD.
  2. Taking moment about A.
  3. find the force in cable B.
  4. using the force and stress, find the area of cable.
  5. Finally using area find the diameter of cable.

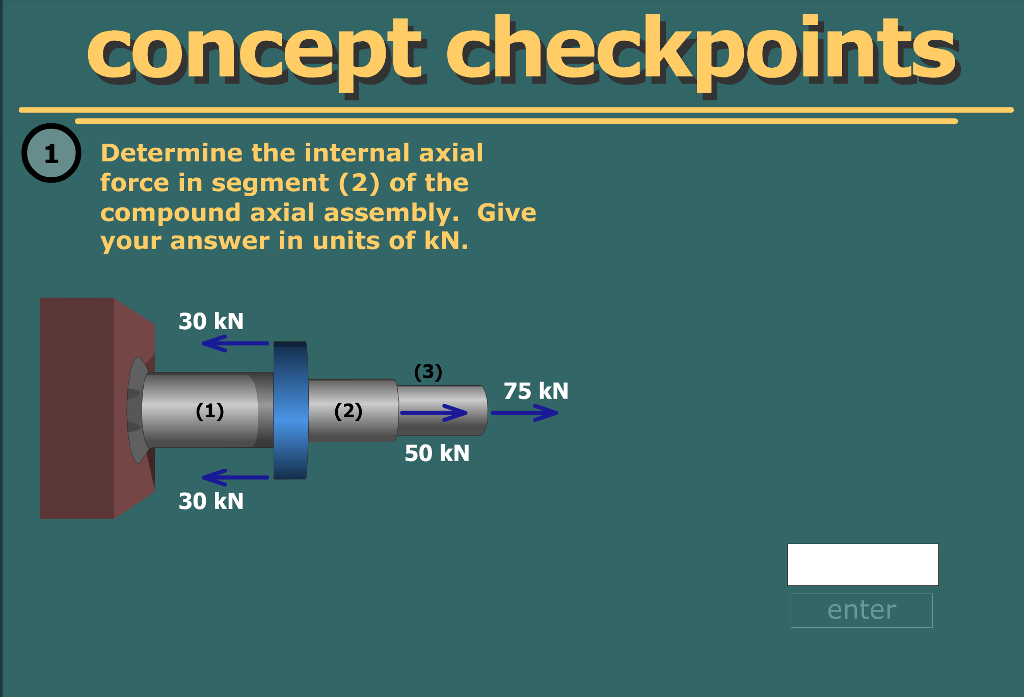
CALCULATE THE INTERNAL FORCE AT SECTION X-X

<Lesson 1-1 HW Fundamental Problem 1.11 5 of 5 -Part A The diamoter of oach segment is ind cated in tho figuro bolaw. Tako P-3 kip,?-9 kip-P3 . 8 kip , and Ps-2 kip (Figure 1) Determine the average normal stress developed at point A. Express your answer to three significant figures and include the appropriate units. Assume negative value in the case of compression and positive value in the case of tension AValue Units Submit Part B Detemine the average normal stress developed at point B Express your answer to three significant figures and include the appropriate units. Assume negative value in the case of compression and positive value in the case of tension I Value Units Submit Request Answer Part C Figure 1 of1 Determine the average normal stress developed at pcint C Expross your answer to three significant figures and include the appropriato units. Assume negative value in the case of compression and positive value in the case of tension 1 in. 0.5 in. 0.5 in. Value Units Submit K Return to Assignment Provide Feedback

Steps to solve:

  1. Draw FBD
  2. from equilibrium find unknown force F.
  3. From the FBD find the force at Section X-X



Posted by at No comments:

ENVIRONMENTAL ENGINEERING

CALCULATE THE HYDRAULIC RETENTION TIME, IF THE VOLUME AND DISCHARGE THROUGH REACTOR IS GIVEN

What is the hydraulic retention time for a factor that has a flow rate of 450 liter per minute and a volume of 32.5m^2

Steps to solve:

  1. HRT is the ratio of volume of the reactor to the discharge through reactor.
  2. convert the discharge from litres per day to cumecs.
  3. now find the HRT 

CALCULATE THE BOD OF WASTE WATER 8ml, FOR WHICH INITIAL AND FINAL DO IS GIVEN

Steps to solve:

  1. Calculate the dilution factor
  2. Now find BOD.



CALCULATE THE DO OF WATER, WHEN WATER FROM FACTORY AND RIVER IS MIXED


Posted by at No comments:

OPEN CHANNEL FLOW

SHOW THAT FOR RECTANGULAR SECTION WIDTH IS TWO TIMES OF DEPTH

Steps to solve:

  1. find perimeter of section in terms of width and depth.
  2. Now convert the perimeter in terms of depth only.
  3. for optimum, perimeter should be minimum.
  4. for minimum differentiate perimeter with respect to depth(y)
  5. from the above relation width is 2 times the depth(y) 



Posted by at No comments:

FRAME STRUCTURE

CALCULATE THE REACTIONS OF THE FRAME

Steps to solve:

  1. Draw FBD.
  2. write equilibrium equation in X and Y directions
  3. Take moment about A.
  4. Now find the reactions at A and B


CALCULATE THE REACTIONS OF THE FRAME

Steps to solve:

  1. Draw FBD.
  2. write equilibrium equation in X and Y directions
  3. Take moment about A.
  4. Now find the reactions at A and B

CALCULATE THE REACTIONS OF THE FRAME

Steps to solve:

  1. Draw FBD.
  2. write equilibrium equation in X and Y directions
  3. Take moment about A.
  4. also take moment about C from left side of C.
  5. Now calculate the reactions at A and B



CALCULATE THE REACTIONS OF THE FRAME

Steps to solve:

  1. Draw FBD.
  2. write equilibrium equation in X and Y directions
  3. Take moment about A.





Posted by at No comments:

TORSION OF SHAFT

CALCULATE THE DIAMETER OF SOLID SHAFT, IF THE ALLOWABLE STRESS AND APPLIED POWER IS GIVEN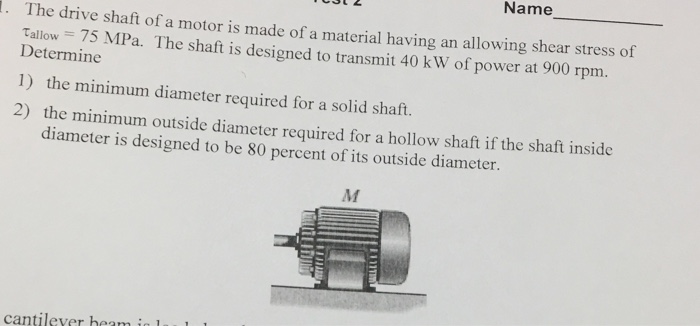Name The drive shaft of a motor is made of a material having an allowing shear stress of Determine 1) the minimum diameter required for a solid shaft. Tallow 75 MPa. The shaft is designed to transmit 40 k W of power at 900 rpm. m outside diameter required for a hollow shaft if the shaft inside diameter is designed to be 80 percent of its outside diameter. cantilever heam i l1


Steps to solve:

  1. Allowable stress, power and rotation is given
  2. from power and rotation find torque
  3. finally find the diameter of shaft

IF THE INTERNAL DIAMETER IS .8 TIMES THE OUTSIDE DIAMETER THEN FIND THE ENTERTAINMENT OF HOLLOW SHAFT

Steps to solve:

  1. Allowable stress, power and rotation is given
  2. from power and rotation find torque
  3. finally find the diameter of shaft


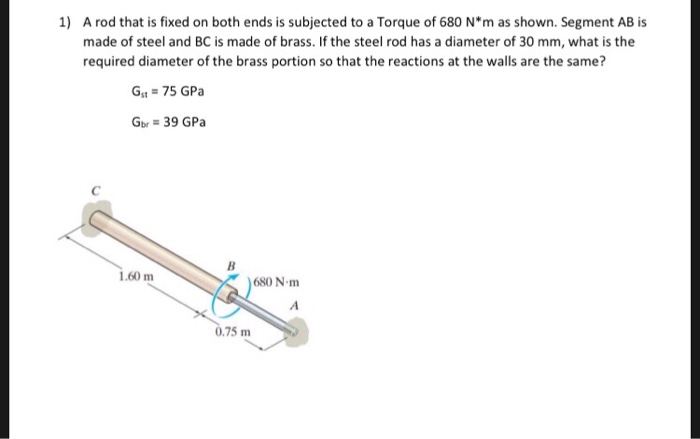
CALCULATE THE DIAMETER OF SHAFT WHEN THE TORSION IS APPLIED

Problem 11.43 3 of 3 The two pulleys attached to the shaft are loaded as shown in the figure below. (Figure 1) VPart A If the bearings at A and B exert only vertical forces on the shaft, determine the required diameter of the shaft using the maximum-shear-stress theory. Suppose that Tallow 12.2 ksi Express your answer to three significant figures and include the appropriate units Figure 1 of 1 dValue Units 0.5 ft Submit Request Answer 1 ft 3 ft 2 ft 120 lb 300 lb 300 lb 120 lb < Return to Assignment Provide Feedback

Steps to solve:

  1. Draw torque diagram in terms of Ta and Tb.
  2. since A and B are fixed hence rotation will be zero
  3. From Above relation find Ta
  4. draw the torque diagram
  5. Now find the diameter of shaft



CALCULATE THE VALUE OF 'P' AND LENGTH OF SHAFT AB



Steps to solve:

  1. Draw toque diagram in terms of P
  2. find polar moment of inertia
  3. from polar moment of inertia find the torue
  4. find the P
  5. find the length AB


1) A rod that is fixed on both ends is subjected to a Torque of 680 N*m as shown. Segment AB is made of steel and BC is made of brass. If the steel rod has a diameter of 30 mm, what is the required diameter of the brass portion so that the reactions at the walls are the same? Gst 75 GPa Gbr 39 GPa 1.60 m 680 N-m 0.75 m
olour Page so ? o, 13 30Gr anan Ct) TOY Steel 9? o. 32. a g


Posted by at No comments:

HIGHWAY ENGINEERING

CHARACTERISTICS OF ROAD TRANSPORT


CALCULATE THE MAXIMUM DENSITY OF ROAD






Posted by at No comments:

STATICALLY INDETERMINATE BEAM

CALCULATE THE REACTIONS OF STATICALLY INDETERMINATE BEAM



CALCULATE THE REACTIONS OF STATICALLY INDETERMINATE BEAM





CALCULATE THE REACTIONS OF STATICALLY INDETERMINATE BEAM





CALCULATE THE REACTIONS OF STATICALLY INDETERMINATE BEAM AND AREA OF SECTION 1 AND 2




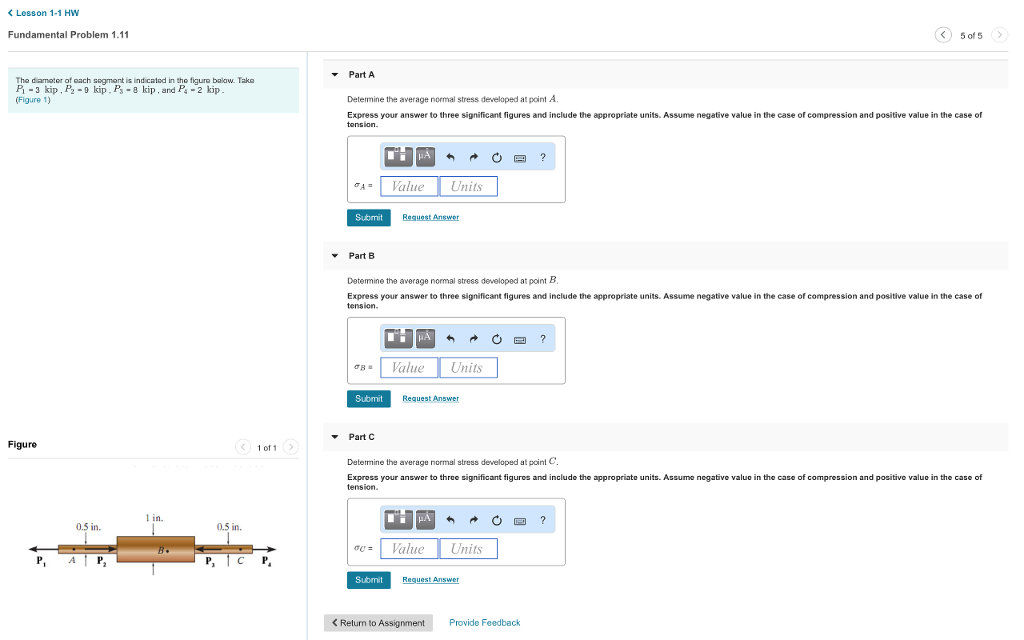
CALCULATE THE STRESS AT POINT A, B AND C











Posted by at No comments:
Subscribe to: Posts (Atom)